The Solution of the Paradox of Achilles and the Tortoise

The Solution of the Paradox of Achilles and the Tortoise

by Giuseppe Gori

In this article I explain Zeno's paradox of Achilles and the tortoise first proposed by Zeno almost twenty five centuries ago. I show what it means to solve a paradox, I propose an explanation of this paradox, and I show why other explanations of this paradox proposed by notable philosophers and mathematicians during the last twenty three hundred years were unsatisfactory.

The History

The paradox of Achilles and the tortoise (one of a set of similar paradoxes) was first introduced by Zeno, a Greek philosopher that lived in the South of Italy approximately 490-450 BC.

According to the procedure proposed by Zeno, Achilles will never reach the tortoise, as every time Achilles reaches the point where the tortoise was, the tortoise has moved further ahead.

The paradox is described, for example, at: https://en.wikipedia.org/wiki/Zeno%27s_paradoxes

This is not a difficult problem to solve, and it is easy to calculate the time it will take for Achilles to reach the tortoise. However, by following Zeno's reasoning the problem seems unsolvable. Thus we have a paradox: two different results for the same problem, depending on which procedure we use.

The paradox has remained unexplained until now.

From the same Wikipedia source we can learn about some of the proposed explanations:

• Aristotle (384 BC−322 BC) remarked that as the distance decreases, the time needed to cover those distances also decreases, so that the time needed also becomes increasingly small.
  Aristotle also distinguished "things infinite in respect of divisibility" (such as a unit of space that can be mentally divided into ever smaller units while remaining spatially the same) from things (or distances) that are infinite in extension ("with respect to their extremities").
• Before 212 BC, Archimedes had developed a method to derive a finite answer for the sum of infinitely many terms that get progressively smaller. Modern calculus achieves the same result, using more rigorous methods. These methods allow the construction of solutions based on the conditions stipulated by Zeno, i.e. the amount of time taken at each step is geometrically decreasing.
• The ideas of Planck length and Planck time in modern physics place a limit on the measurement of time and space, if not on time and space themselves.
• According to Hermann Weyl, the assumption that space is made of finite and discrete units is subject to a further problem, given by the "tile argument" or "distance function problem"
• Infinite processes remained theoretically troublesome in mathematics until the late 19th century.
  While mathematics can calculate where and when the moving Achilles will overtake the Tortoise of Zeno's paradox, philosophers such as Brown and Moorcroft claim that mathematics does not address the central point in Zeno's argument, and that solving the mathematical issues does not solve every issue the paradoxes raise.
• Debate continues on the question of whether or not Zeno's paradoxes have been resolved. In The History of Mathematics: An Introduction (2010) Burton writes, "Although Zeno's argument confounded his contemporaries, a satisfactory explanation incorporates a now-familiar idea, the notion of a 'convergent infinite series.' ".
• Bertrand Russell offered a "solution" to the paradoxes based on the work of Georg Cantor, but Brown concludes "Given the history of 'final resolutions', from Aristotle onwards, it's probably foolhardy to think we've reached the end.

From: https://plus.maths.org/content/mathematical-mysteries-zenos-paradoxes (A mathematics magazine):

• “So Zeno's paradoxes still challenge our understanding of space and time, and these ancient arguments have surprising resonance with some of the most modern concepts in science.”

From: https://www.jstor.org/stable/20833062?seq=1#page_scan_tab_contents:

• “In his Lectures on the History of Philosophy (first delivered in 1805-6) Hegel said that "Zeno’s dialectic of matter has not been refuted to the present day: even now we have not got beyond it, and the matter is left in uncertainty."
• Bertrand Russell, in his “Recent work in the Philosophy of Mathematics", written in l90l, held that “From him [i.e. Zeno] to our own day, the finest intellects of each generation in turn attacked the problems, but achieved, broadly speaking, nothing."
  Russell, however, thought that the problems involved in Zeno’s paradoxes, which he identified as "the problems of the infinitesimal, the infinite, and continuity”, have not merely been advanced by the mathematicians of his own age but have been completely solved.
• Some philosophers, notable among them Bergson, refused right from the beginning to accept the newly-advanced theories of continuity and infinity and the consequent definition of motion, and endeavored to offer alternative definitions of motion, continuity and infinity. Since the mathematical solution found a number of champions, including no less able an advocate than Russell, and there was no dearth of the defenders of the philosophic tradition, there ensued a battle royal which brought to the fore scores of re-interpretations of the paradoxes and as many 'solutions'.
• No solution, however, was found to be tenable, and soon philosophers despaired of finding a solution that would be acceptable to all.
• In the 1950s, Prof. Ryle, in offering a solution of the Achilles-Tortoise paradox, feared that the fate of his solution would be, like that of his predecessors’ solutions, "demonstrable failure”, and, Prof. Lazerowitz went to the extent of opining that the paradoxes are (valid) theorems of some metaphysical wish-fulfillment language.
• The position as it then obtained, (that is, philosophers and mathematicians inability to overtake Zeno) was ably summed up by Prof. Owen in his masterly paper of 1957-58.
• Many a solution has appeared since then, including one by Shamsi, the author of the artice, but none succeeds in resolving the paradoxes as a whole.”

What Does Solving a Paradox Mean?

As mentioned at the beginning of this article, a paradox proposes the existence of two different results as a solutions for the same problem. These results are inconsistent with each other, depending on which procedure is used. Only one can be correct.

As Brown and Moorcroft suggest, we are not looking for a mathematical demonstration that Achilles reaches the tortoise. Assuming they are both running in the same direction, we know he will. We can calculate the exact time, given the distance between the two and the two speeds, using a simple formula:

t = distance/(difference in velocity)

Instead, explaining or invalidating a paradox is to show a fault in the paradox formulation, or the proposed solving procedure, so that we can exclude this procedure and demonstrate that there is only one result for the original problem.

The solution of a paradox is the answer to the question: “How does the paradox formulation misrepresent reality or logic?” That is, we need to show why the proposed method is conceptually wrong.

Solving a paradox, invalidates the formulation of a problem proposed by the author of the paradox and leaves us with only valid procedures for solving the original problem. You can read: “Solving a Paradox, What does it Mean?” at: https://bit.ly/2IudnVO

Why were the previous proposed solutions for the paradox not satisfactory?

The fault of most, if not all, the proposed solutions to Zeno's paradox is the assumption that Zeno's proposed procedure was correct. The procedure seems to be logical when it is first introduced to us, but we will see that the procedure proposed by Zeno is deceptive.

The authors then tried using Zeno's deceptive procedure to reach the expected correct result for the original problem.

The Explanation of Zeno's Paradox:

Zeno's proposition invites the solver to do a series of steps each time changing system of reference:

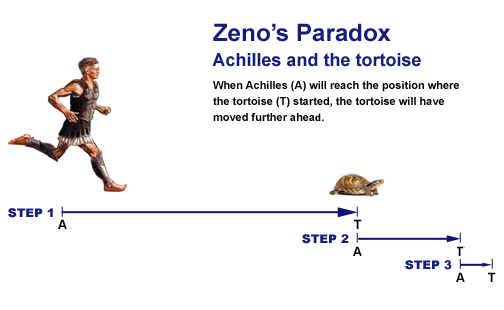
STEP 1: The starting system of reference: The point where Achilles starts the race and the tortoise is well ahead,

STEP 2: After a while, we are then asked to use a new system of reference: The point where Achilles reached and where the tortoise initially started, with the the tortoise now a bit further ahead,

STEP 3: Then again we are asked to use, recursively, a new system of reference with the new starting point for Achilles and with the tortoise still further ahead,

with every step we are asked to freeze the process and then continue by re-creating and examining the original problem using a different system of reference.

After Zeno's proposed first step, or first change of system of reference, the problem, as presented in the second step, is exactly the same as the original, the only change being a difference in "scale". No progress was achieved in solving the problem.

Changing system of reference essentially restarts the problem-solving procedure.

This realization implies that the problem is never going to reach a conclusion as the step by step procedure is reiterated.

If the system of reference is changed at every step, our working spacetime shrinks with every step, the solution becomes elusive and the tortoise becomes apparently unreachable.

Zeno proposes a procedure that never ends, for solving a problem that has a trivial solution.

A Programming Analogy

Zeno's proposed procedure is analogous to solving a problem by recursion, a well known problem solving technique available in modern programming languages. However, recursion would be the wrong technique to solve the original problem.

If the problem was programmed exactly as Zeno suggested, the program would never end normally, simply because the condition for the end of the recursion process (Achilles reaches the tortoise) would never occur. Our computer program would confirm the paradox!

Our Solution of Zeno's Paradox

Our solution of Zeno's paradox can be summarized by the following statement:

"Zeno proposes observing the race only up to a certain point, using a system of reference, and then he asks us to stop and restart observing the race using a different system of reference. This implies that the problem is now equivalent to the original and necessarily implies that the proposed procedure for solving the problem will never end."

That’s it. You cannot change system of reference in the middle of a problem that uses a system of reference, whether openly stated, or implied.

As an analogy, you cannot solve a problem involving measurements by using English Imperial measures at the start of calculations and then switch to metric measures (without proper conversions) in the middle of calculations.

In another similar paradox, the Dichotomy paradox, Zeno uses the same argument to paradoxically state that we cannot go from point A to point B, because it would take an infinite number of steps and we would never arrive. In this case as well, we can show that the proposed method involves changing the system of reference in the middle of the proposed procedure. Thus the proposed method for solving the problem is invalid.

An example

The following is not a “solution” of the paradox, but an example showing the difference it makes, when we solve the problem without changing the system of reference.

In this example, the problem is formulated as closely as possible to Zeno’s formulation.

Zeno would agree that Achilles makes longer steps than the tortoise.

Let’s assume that one Achilles-step is about 20 tortoise-steps long, and let’s also assume that both Achilles and the tortoise make the same number of steps in the same amount of time. For example, two steps per second (the exact amount doesn’t really matter).

If the tortoise starts the race 20 Achilles-steps ahead of him, then after 20 steps Achilles reaches where the tortoise was (See diagram below: Tortoise starting point).

In the meantime, the tortoise has made 20 of her steps, and she is now one full Achilles-step ahead of him.

We have not changed our system of reference. We referred to both starting points. These did not move relatively to each other. We could choose any fixed ground point. To please Zeno, let’s continue by referring to the tortoise starting point, where Achilles currently is.

When both runners make one more step, step 21, the tortoise will have moved by one of her steps and she will still be ahead of Achilles by that one tortoise-step. Achilles is now one Achilles-step ahead of the tortoise starting point.

Now, let’s continue, without changing the system of reference. This is the key point.

We do not redefine the problem and use the current positions of the runners as new starting points, as Zeno proposes, but we refer to the information about the race we have already accumulated in our knowledge base.

Achilles then completes his 22^nd step, and he is two Achilles-steps ahead of the tortoise starting point. The tortoise will have completed her 22^nd tortoise-step from her starting point. Hence the tortoise is now behind Achilles by 18 tortoise-steps.

Thus, if we do not change the system of reference, the paradox does not appear.

The Dichotomy paradox

In another similar paradox, the Dichotomy paradox, Zeno uses the same argument to paradoxically state that we cannot go from point A to point B, because it would take an infinite number of steps and we would never arrive. In this case as well, we can show that the proposed method involves changing the system of reference in the middle of the proposed procedure. Thus the proposed method for solving the problem is invalid.